Python中关于set的基本用法

目录

• 1. set 的基本内容
• 1.基本特点
• 2.set() 实质
• 2. set 的基本方法
• 2.1 set 的普通基本方法
• 2.2 set 的逻辑基本方法
• 总结

1. set 的基本内容

1.基本特点

• (1) 无序性
• (2) 确定性
• (3) 不重复性

2.set() 实质

内部进行 可迭代性的 for 循环

例子：

2. set 的基本方法

2.1 set 的普通基本方法

2.1.1 增

add(self, *args, **kwargs)
copy(self, *args, **kwargs)

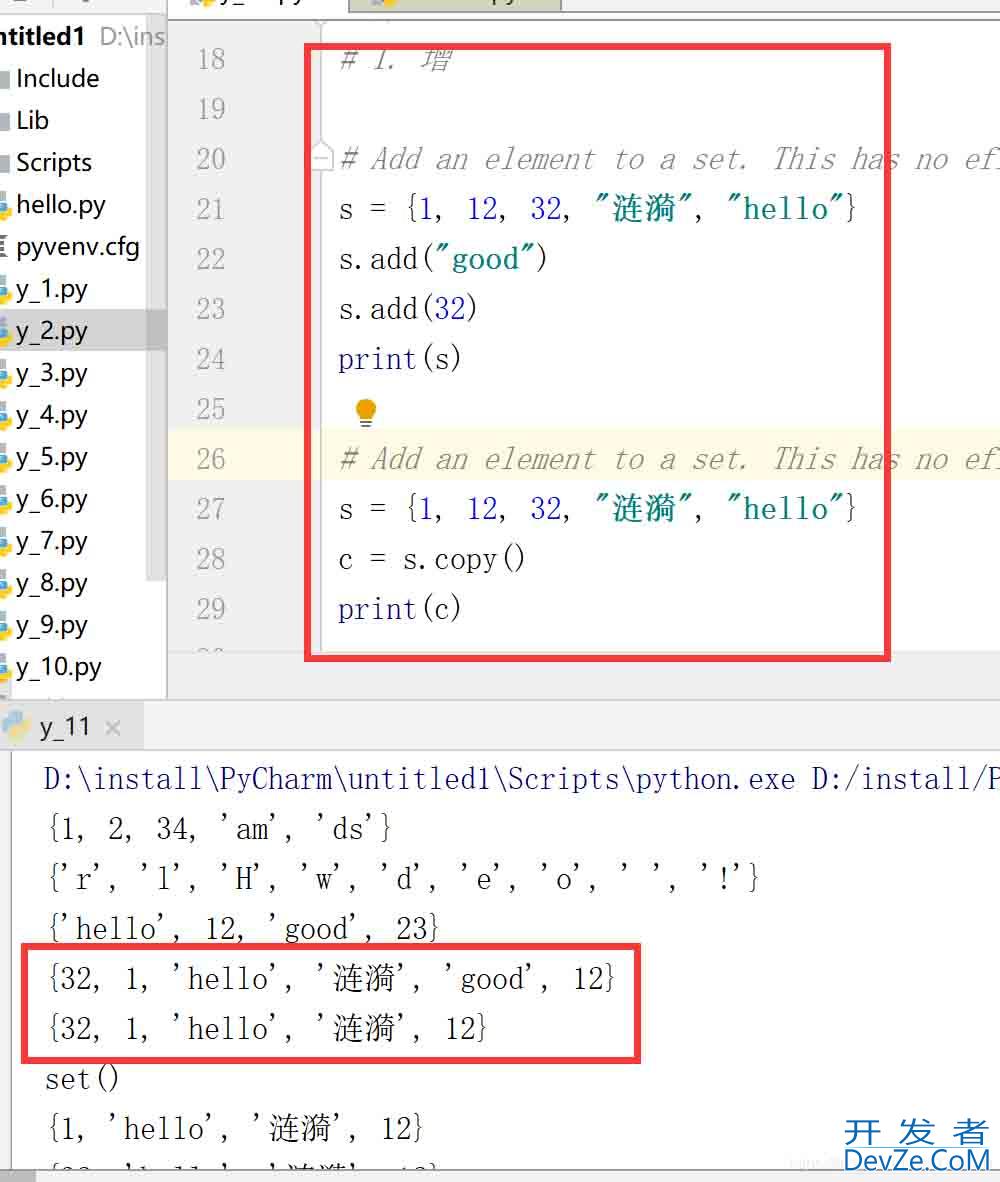
# 1. 增

# Add an element to a set. This has no effect if the element is already present.
s = {1, 12, 32, "涟漪", "hello"}
s.add("good")
s.add(32)
print(s)

# Add an element to a set. This has no effect if the element is already present.
s = {1, 12, 32, "涟漪", "hello"}
c = s.copy()
print(c)

结果：

2.1.1 删

clear(self, *args, **kwargs)
pop(self, *args, **kwargs)
remove(self, *args, **kwargs)
discard(self, *args, **kwargs)

# 2. 删

# Remove all elements from this set.
s = {1, 12, 32, "涟漪", "hello"}
s.clear()
print(s)

# Remove and return an arbitrary set element. Raises KeyError if the set is empty.
s = {1, 12, 32, "涟漪", "hello"}
s.pop()
print(s)

# Remove an element from a set; it must be a member. If the element is not a member, raise a KeyError.
s = {1, 12, 32, "涟漪", "hello"}
s.remove(1)
# s.remove("good")
print(s)

# Remove an element from a set if it is a member. If the element is not a member, do nothing.
s = {1, 12, 32, "涟漪", "hello"}
s.discard(1)
s.discard("good")
print(s)

结果：

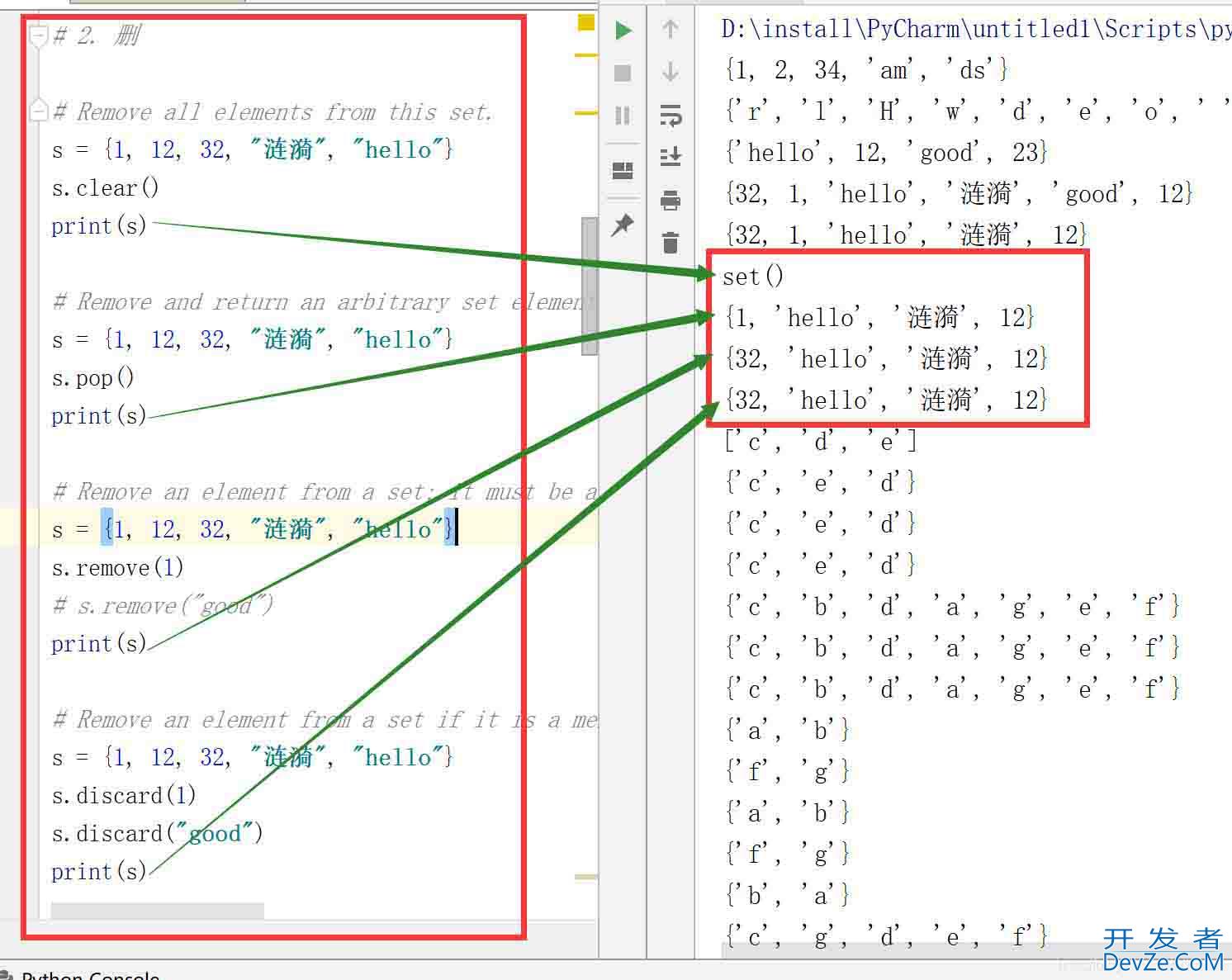
pop() 是随机删除。

remove() 和 discard() 指定删除，但是指定不存在的元素时，remove() 会报错，而 discard() 不会报错

2.2 set 的逻辑基本方法

2.2.1 set 交集运算

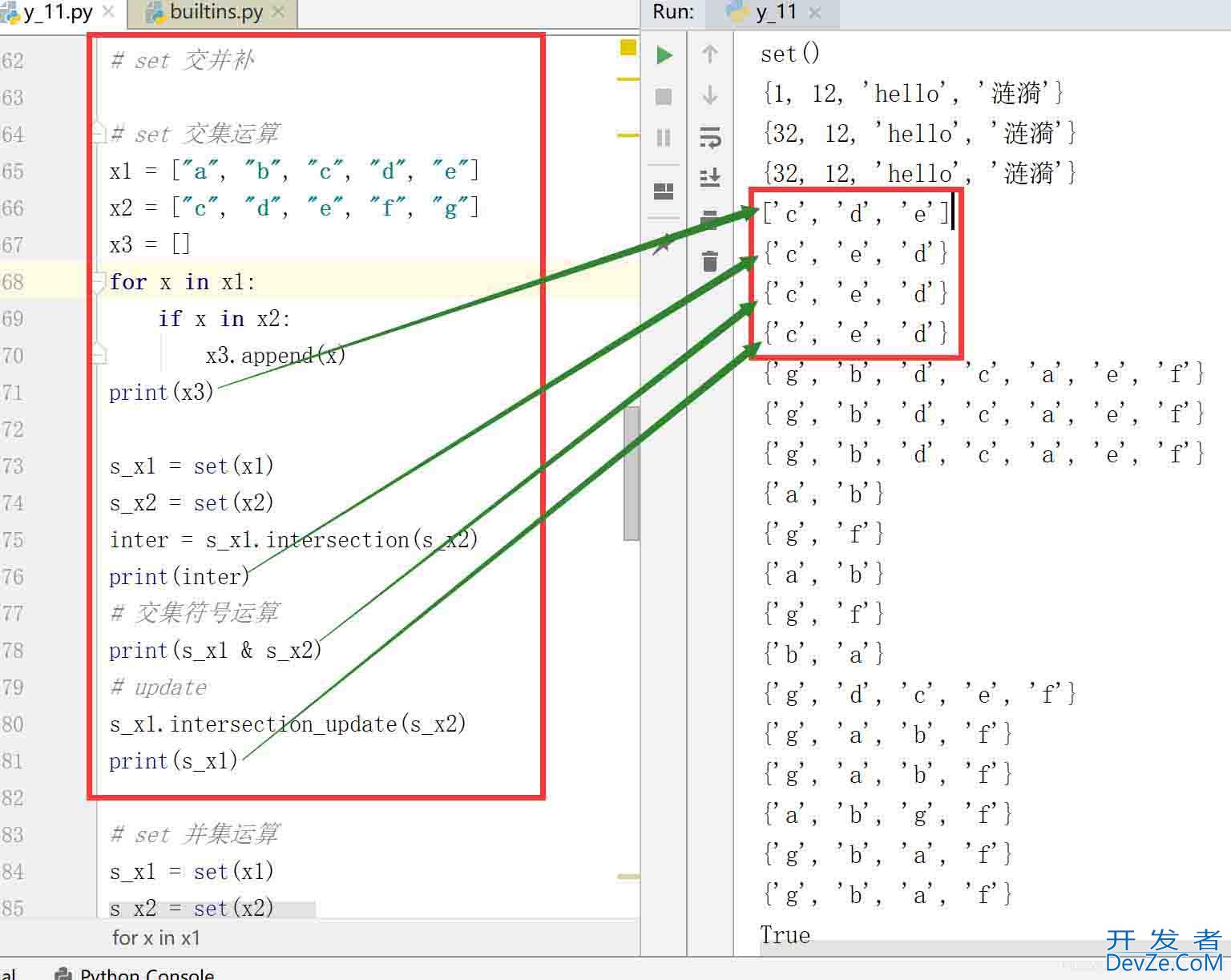
# set 交集运算
x1 = ["a", "b", "c", "d", "e"]
x2 = ["cDquKVKzGHA", "djavascript", "e", "f", "g"]
x3 = []
for x in x1:
    if x in x2:
        x3.appepythonnd(x)
print(x3)

s_x1 = set(x1)
s_x2 = set(x2)
inter = s_x1.intersection(s_x2)
print(inter)
# 交集符号运算
print(s_x1 & s_x2)
# update
s_x1.intersection_update(s_x2)
print(s_x1)

结果：

2.2.2 set 并集运算

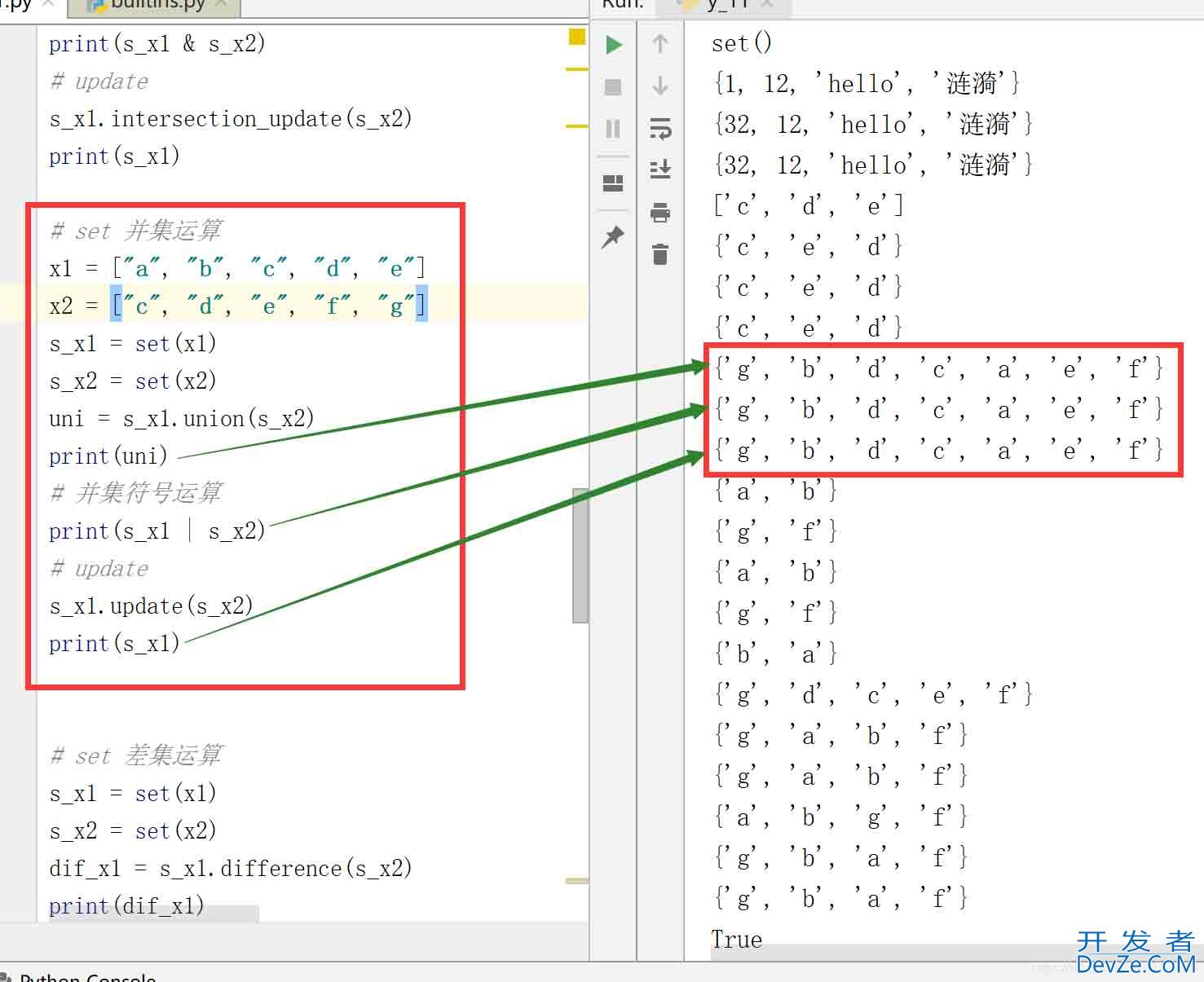
# set 并集运算
x1 = ["a", 编程客栈"b", "c", "d", "e"开发者_JAVA教程]
x2 = ["c", "d", "e", "f", "g"]
s_x1 = set(x1)
s_x2 = set(x2)
uni = s_x1.union(s_x2)
print(uni)
# 并集符号运算
print(s_x1 | s_x2)
# update
s_x1.update(s_x2)
print(s_x1)

结果：

2.2.3 set 差集运算

# set 差集运算
x1 = ["a", "b", "c", "d", "e"]
x2 = ["c", "d", "e", "f", "g"]
s_x1 = set(x1)
s_x2 = set(x2)
dif_x1 = s_x1.difference(s_x2)
print(dif_x1)
dif_x2 = s_x2.difference(s_x1)
print(dif_x2)
# 差集符号运算
print(s_x1 - s_x2)
print(s_x2 - s_x1)
# update
s_x1.difference_update(s_x2)
print(s_x1)
s_x2.difference_update(s_x1)
print(s_x2)

结果：

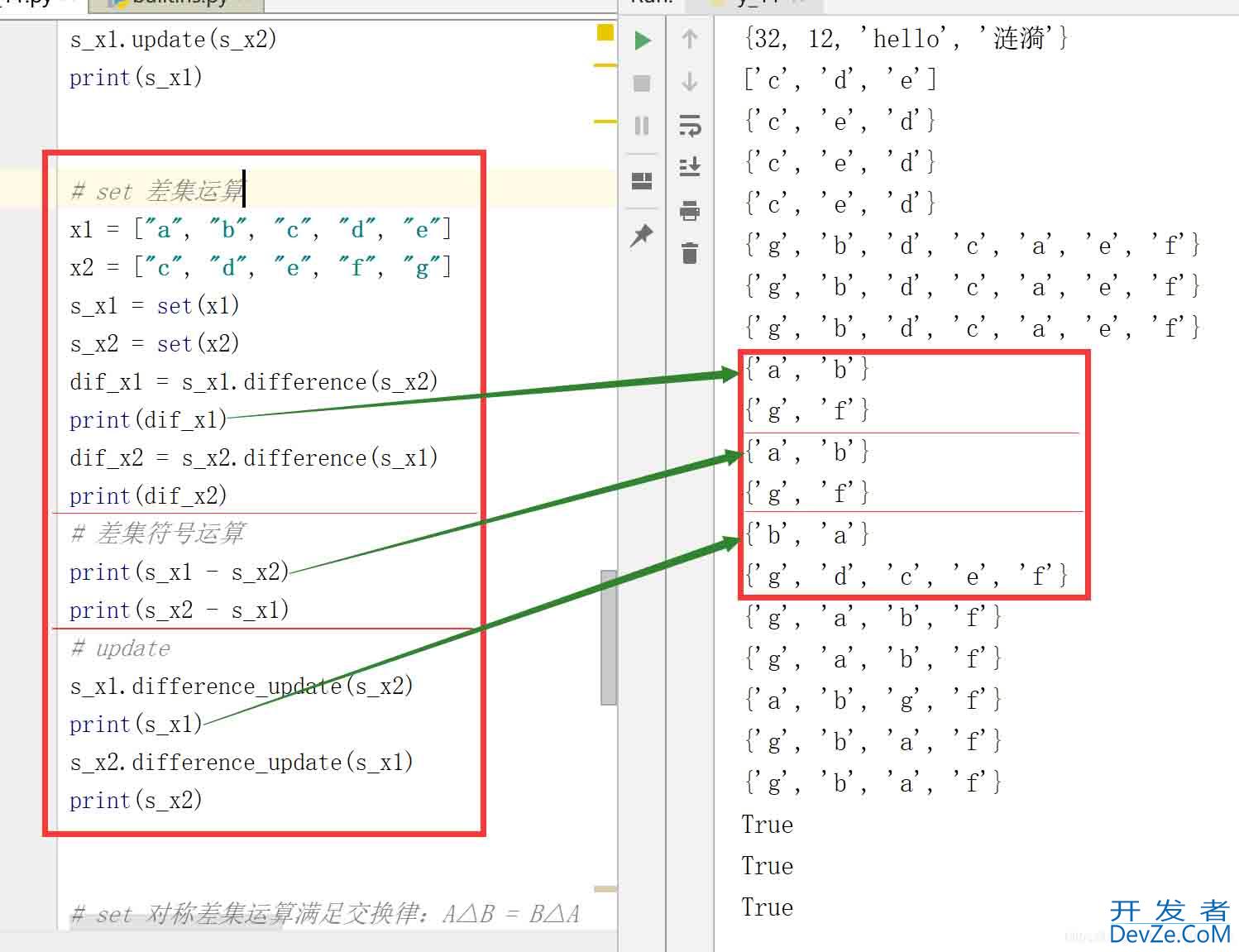
2.2.4 set 对称差集运算

# set 对称差集运算满足交换律：A△B = B△A
s_x1 = set(x1)
s_x2 = set(x2)
sym = s_x1.symmetric_difference(s_x2)
print(sym)
# 对称差集符号运算
print(s_x1 ^ s_x2)
print(s_x1 - s_x2 | s_x2 - s_x1)
print((s_x1 | s_x2) - (s_x2 & s_x1))
# update
s_x1.symmetric_difference_update(s_x2)
print(s_x1)

结果：

2.2.5 set 逻辑判断运算

# 判断
# Return True if two sets have a null intersection.
x1 = {"a", "b", "c"}
x2 = {"e", "f", "g"}
inter = x1.isdisjoint(x2)
print(inter)

# Report whether another set contains this set.
x1 = {"a", "b", "c"}
x2 = {"a", "b", "c", "e", "f", "g"}
inter = x1.issubset(x2)
print(inter)

# Report whether this set contains another set.
x1 = {"a", "b", "c", "e", "f", "g"}
x2 = {"a", "b", "c"}
inter = x1.issuperset(x2)
print(intejsr)

结果：

总结

以上为个人经验，希望能给大家一个参考，也希望大家多多支持我们。